DO YOU KNOW THAT...
- The name for zero is not settled even yet. Older names and variations include naught, tziphra, sipos, tsiphron, rota, circulus, galgal, theca, null, and figura nihili.

- Thomas Harriot (1560–1621), an English mathematician, was the first to make use of these symbols: ‘>‘ for ‘is greater than’ and ‘<‘ for ‘is less than’.

- The Anglo-American symbol for division is of 17 th century origin, and has long been used on the continent of Europe to indicate subtraction. Like most elementary combinations of lines and points, the symbol is old. It was used as early as the 10th century for the word est. When written after the letter ‘I’, it symbolized ‘id est’. When written after the word ‘it’, it symbolized ‘interest’.  If written after the word ‘divisa’, for ‘divisa est’, this might possibly have suggested its use as a symbol of division. Towards the close of the 15th century the Lombard merchants used it to indicate a half, along with similar expressions such as this one
If written after the word ‘divisa’, for ‘divisa est’, this might possibly have suggested its use as a symbol of division. Towards the close of the 15th century the Lombard merchants used it to indicate a half, along with similar expressions such as this one 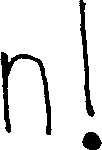 on the right.
on the right.
- The symbol n! for ‘factorial n, now universally used in algebra, is due to Christian Kramp (1760–1826) of Strassburg, who used it in 1808.
-  Our familiar signs, in geometry, for similar and for congruent are
Our familiar signs, in geometry, for similar and for congruent are  due to Leibniz (1646–1715.)
due to Leibniz (1646–1715.)
- This symbol for pi was used by the early English mathematicians William Oughtred (1574–1660), Isaac Barrow (1630–1677), and David Gregory (1661–1701) to designate the circumference (or periphery) of a circle. The first to use the symbol for the ratio of the circumference to the diameter was the English writer, William Jones, in a publication in 1706. The symbol was not generally used in this sense, however, until Euler (1707–1783) adopted it in 1737.
- In 1923, the National Committee on Mathematical Requirements, sponsored by the Mathematical Association of America, recommended this symbol (on the left) as standard usage for angle in the United States. Historically, Pierre Herigone, in a French work in 1634, was apparently the first person to use a symbol for angle. He used both the symbol above as well as this symbol on the right, which had already been used to mean ‘less than.’ The standard symbol survived, along with other variants, as follows.
V. Find some information on the history of the mathematical symbols. Give a presentation to the students of your group.
Text 4
Reading and Speaking
STATISTICS
The term statistics is ultimately derived from the New Latin ‘statisticum collegium’ (‘council of state’) and the Italian word ‘statista’ (‘statesman‘ or ‘politician‘). The German word ‘Statistik’, first introduced by Gottfried Achenwall (1749), originally designated the analysis of data about the state, signifying the ‘science of state’ (then called political arithmetic in English). It acquired the meaning of the collection and classification of data generally in the early 19th century. It was introduced into English in 1791 by Sir John Sinclair when he published the first of 21 volumes titled ‘Statistical Account of Scotland’.
In the 18th century the term ‘statistics‘ designated the systematic collection of demographic and economic data by states. In the early 19th century, the meaning of this term was broadened, then including the discipline concerned with the collection and analysis of data. Today statistics is widely employed in government, business, and all the sciences. Electronic computers have expedited statistical computation, and have allowed statisticians to develop ‘computer-intensive’ methods.
The term ‘mathematical statistics’ designates the mathematical theories of probability and statistical inference, which are used in statistical practice. In the 19th century, statistics increasingly used probability theory, the initial results of which were found in the 17th and 18th centuries, particularly in the analysis of games of chance (gambling). In the 18th century astronomy used probability models and statistical theories, particularly the method of least squares, which was invented by Legendre and Gauss. Early probability theory and statistics were systematized and extended by Laplace; following Laplace, probability and statistics have been in continual development. In the 19th century, social scientists used statistical reasoning and probability models to advance the new sciences of experimental psychology and sociology; physical scientists used statistical reasoning and probability models to advance the new sciences of thermodynamics and statistical mechanics. The development of statistical reasoning was closely associated with the development of inductive logic and the scientific method.
Statistics is not a subfield of mathematics but an autonomous mathematical science, like computer science. Unlike mathematics, statistics had its origin in public administration and maintains a special concern with demography and economics. Being concerned with the scientific method and inductive logic, statistical theory has close association with the philosophy of science; with its emphasis on learning from data and making best predictions, statistics has great overlap with the decision science and microeconomics. With its concerns with data, statistics has overlap with information science and computer science.
Today the use of statistics has broadened far beyond its origins. Individuals and organizations use statistics to understand data and make informed decisions throughout the natural and social sciences, medicine, business, and other areas. Many universities maintain separate mathematics and statistics departments. Statistics is also taught in departments as diverse subject as psychology, education, and public health.
I. Match the words (1–5) with their definitions/explanations (a–e):
| to designate | a | to help forward, to speed up | |
| to derive from | b | to mean | |
| to signify | c | to have as a source or origin | |
| to expedite | d | to give name to | |
| reasoning | e | the process of reaching conclusions by using one’s reason |
Дата: 2016-10-02, просмотров: 337.